Algebra problem from hell

What were you thinking?

The best student in my elementary algebra class looked up from the last page of the exam and tried to kill me with her eyes. It startled me. Surely, of all people, she should have taken it in stride. She picked up her exam and came forward to see me. “We never did these,” she said.

I understood. That exact problem had not appeared in either the homework or any of the quizzes. She seriously judged the exercise to be out of bounds. I had betrayed their trust.

How egregious was my crime? Consider: The exam was on systems of linear equations. All of the problems were limited to two equations with two unknowns. (This is elementary algebra, after all.) We had solved systems by graphing the lines and checking the results by substitution (just a glorified version of “guess & check”—and limited to cases with really simple solutions). We had solved systems by solving one equation for a variable and substituting the result into the second equation. Finally, we had solved systems by adding the equations (or their equivalents) so that one of the variables was eliminated.

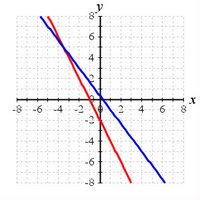My students were dismayed that the last problem on the exam was not precisely any of these. Instead I had presented them with a graph that displayed two lines:

Find the equations of the two lines in the figure and then solve the corresponding system of equations to determine where they cross.

---

This problem turned into one of the starkest discriminators ever between my strongest and weakest students. The best student in class, who had forthrightly expressed her dismay, calmed down as soon as I asked her if she knew how to find the equation of a line. “Yes, sure, if I know some points on the line.” “Well,” I said, “I've given you lots to pick from if you can read a graph.”

We had read many graphs in the previous chapters on linear functions and equations. We had worked lots of problems involving finding slopes and equations of lines. I was expecting them to remember how all of that work was related to the content of the current chapter, especially since we had never stopped doing such exercises. A few students just gave up. A few more tried to read the intersection point from the graph but abandoned any attempt to find the actual equations of the system. (They got a few points if they correctly read the intersection coordinates.) A handful realized that they knew everything they needed to solve the problem in detail. Both lines intersected several grid nodes. (I wonder what would have occurred if I had highlighted those points as in the accompanying figure.)

After I had graded the exam, I noticed that the infamous exercise had turned into something very close to an all-or-nothing situation for most of my students. Either they received a meager number of points for their futile (or nonexistent) efforts or they raked in virtually all of the points possible on the problem. Only six managed an A on the problem, while three were at the D level and twenty-five bombed it outright. The table shows how the students who earned 90% or more of the credit on the crossed-lines problem tended to be A students. When I checked my records for the one exception—the C student—I discovered that he was a chronic class-cutter. He seemed to know his stuff but had missed almost all the quizzes and taken a big hit on his course grade because of his lower overall point total. In other words, high performance on this one problem was a sure indicator in this class of high-level algebra skills. However, it simply split the class into two camps with no gradations in between: the A students versus everyone else. As I said before in a slightly different context, it was essentially all or nothing. (Of course, we should note that the problem grade and the course grade are hardly independent of each other since the latter incorporates the points earned on the former. Still, by this point in the semester a single problem on one exam has only a small effect on the cumulative grade.)

My students and I reviewed all the problems and their solutions when I returned the exam. Some of them commented on how similar the problem was—in retrospect—to earlier quiz and exam problems where they had been required to compute slopes by reading points from the coordinate grid. I won't claim that they were happy about it, but they had at least recognized that the problem was not as shockingly new as they had originally thought. Now they're expecting a follow-up quiz that will give them an opportunity to earn points by demonstrating they learned the lesson of the algebra problem from hell. I won't disappoint them and I hope that they won't disappoint me.

Sic transit

A look backward

I broke a link the other day. I hadn't meant to break it. More likely, it was already broken and I finally realized it. Sometimes you don't fully comprehend that a connection no longer exists until long afterward. This is such an occasion.

The memorial service was noted on my calendar a number of weeks ago and I fully intended to go. On the day in question, however, there was a flurry of distractions at school, papers to grade, and classes to teach. Although I was heading toward my home town with enough time to make the service, by then I had forgotten about it. Only hours later did I realize what I had neglected.

The memorial was for the last surviving offspring of my former landlady, the only one to share (in some measure) her mother's longevity, although not quite in the same league. My old landlady made it well into her nineties, while her daughter had to be content with reaching the eighties. My connection with my former landlady and her family was forged back in the 1970s, when I came to town to attend graduate school at the local university and began what turned into a residency of several years in the student quarters in her basement.

“Miss Jane” was a widow who had come west with her husband when he took a position at the university. She had the southern-tinged hospitality of a border-state native and never quite seemed comfortable as a Californian. She had been on her own for decades when I met her, but she was comfortably set up with some investments, survivor benefits from the university, and her own pension from service as a university administrative assistant. Many of the buildings on campus bear names that she knew as living people. Miss Jane rented out a room for company's sake and the presence of a young person who might lend the occasional hand when a petite elderly woman needed something from a tall shelf. The rent was a wonderful bargain for me, especially since my landlady's house was close to the university and made commuting almost nonexistent. A short stroll sufficed to get me anywhere on campus.

I gradually discovered that living with Miss Jane had all the advantages—and disadvantages—of living with one's grandmother. She tended to spoil her student residents, sometimes offering meals (not part of the rental agreement) and always inviting me to join her when grandchildren were visiting for dinner. She also had copious amounts of advice and kept close tabs on her residents. Like I said, it was as if you were living with your grandmother.

Although I stayed with her a full year beyond grad school (during a part-time teaching appointment at the university), Miss Jane still seemed a bit miffed when I moved into an apartment at the beginning of the legislative job I obtained in 1979. I continued to visit her on a nearly weekly basis while a succession of new students occupied her basement rental. By then I had a thoroughly ambivalent role to play in Miss Jane's life scenario and family constellation. I was part of the bulwark she had erected against the world.

Miss Jane was a fierce little woman in many ways and often at odds with the community zeitgeist: a conservative Republican in a liberal college town, a semi-southerner in a western state, and a senior citizen in a relentlessly young population. (If you're a teacher, you already know that your students' youth is renewed each year while you keep getting older. Living next door to a university gives you a similar experience.) Miss Jane was not a cheerful person, and the passing years did not give her much reason to become more optimistic. She had already outlived her spouse and one offspring when I met her and would soon outlive another of her children. She seemed always alert and on guard against the further shocks that she expected life to mete out.

As someone who existed inside her defense perimeter, I gradually became one of her trusted few. Miss Jane particularly liked having a resident mathematician who could easily balance her checkbook to the penny every month. It was a simple favor to do for my landlady and soon I took care of it as a matter of course. Miss Jane's surviving daughter had a husband who was more than eager to be helpful with her accounts, but my landlady seemed to enjoy teasing her son-in-law by telling him it was all under control. It was entirely natural that he began to regard the resident grad student as an interloper, especially when I was no longer a resident and Miss Jane continued to save things for me to look at when I would make my next social call. She was using me to maintain a modicum of independence from those who had the actual responsibility to look out for her in her advancing age, but I didn't clearly perceive it that way at the time. I wonder if she did.

After I had been out of Miss Jane's home for a decade and she had to move into a residential-care facility, she had faced the necessity of putting her affairs under the management of her daughter and son-in-law. I was always able to maintain casually friendly relations with Miss Jane's daughter, who gave me some piano music from her mother's collection when it came time to shut down the house. Unlike her husband, the daughter's pride was apparently not pricked by the knowledge that mother preferred to have a “stranger” balance her checkbook.

I last saw Miss Jane's daughter at the drugstore late last year, when we coincidentally arrived at the same time to pick up prescriptions. As I said my name to the clerk, I heard her say her name just as clearly to a different clerk. When I made my purchase and turned toward her to say hello, I saw that she was already walking away with prescription in hand. It was not until I saw the obituary last month that I learned she had become nearly deaf and had vision problems. She hadn't heard my name at all and she probably hadn't even seen me. (Besides, I have changed a little in the fifteen years since I was enmeshed in that family circle.)

The daughter's death notice and memorial service announcement informed me that she had herself recently become a widow. I resolved to go to the service and offer my condolences to her children and the various nieces and nephews—Miss Jane's grandchildren—who might be there. Instead, as you saw, I did not go. How could I forget something that was my last connection to a big chunk of my college life? I guess it was already over. I just didn't know it until now.

Requiescat in pace.

Whoring for jr

Brit has been there before

Reversion to the mean is a phenomenon unhappily familiar to a host of people whose names bear the appendage “Jr.” Just ask the juniors whose names are Frank Sinatra, Hank Williams, or Franklin Roosevelt. When a remarkable talent appears in a family's line of descent, chances are extremely good that the next generation will suffer by comparison. To quote MathWorld, “ [A]n extreme event is likely to be followed by a less extreme event.”

Some people, however, take an extraordinarily long time to recognize when reversion has struck, even when the evidence keeps shoving itself in your face. Brit Hume of Fox News is one such person. It's happened to him at least twice, and both occasions were painfully obvious to disinterested observers. Brit is very good at keeping the faith.

The first time Brit Hume fell at the feet of an unworthy scion of aristocratic descent was in the 1980s. The pedigree could not have been more elevated, but the results could scarcely have been more disappointing. IBM had provided the personal computing industry with overnight credibility when it deigned to enter the market in 1981 with the IBM Personal Computer. For all of its limitations—some of them significant—Big Blue's desktop computer was immensely successful and quickly invaded business offices and homes across the world. After all, the name of IBM conjured digital magic and everyone took it seriously. The pioneering companies in home computing were swept away, with only Apple hanging on as a distant second.

Everyone assumed that the IBM juggernaut would continue with its follow-up products, and to a degree everyone was right. IBM tweaked the PC by adding a hard disk model (the PC XT in 1983) and a larger model with a more powerful microprocessor (the PC AT in 1984), but the real buzz concerned Big Blue's second generation computer—code-named “Peanut.” We who belonged to computer user groups had access to the latest and most lurid rumors: Peanut would be multitasking. Peanut would have a windowing operating system. Peanut would have dazzling graphical capabilities. Peanut would revolutionize personal computing.

The elephant labored mightily and brought forth ... a mouse. The IBM PCjr was an instant anticlimax. The keyboard was an embarrassment (IBM had to redesign and replace it). The graphics were a slight improvement over those of the original PC (more colors, but the same resolution). There was only one disk drive (and it was a floppy, of course). The slots for ROM cartridges had no software except for a few games (and Lotus 1-2-3, the only serious business program ported to the jr). With additional expenditures for add-ons and secondary market products, a user could bring the PCjr up to the level of a regular PC, by which point its modest price advantage relative to the original PC was more than wiped out.

The IBM PCjr was released with great fanfare in 1984 and quietly killed in 1985. Big Blue burned off its large inventory of unsold PCjrs with deep discounts during the 1984 Christmas season. Brit Hume was one of the many people who went for the bait and found themselves cut off when IBM discontinued the product and its support a few months later. He made the best of a bad deal by sharing his observations and travails in articles published in Monitor, the monthly newsletter of the Capital PC User Group in Washington, D.C. Brit's column carried various titles, but the most common one was Living with jr. His running theme was that the jr wasn't that bad. Really.

Once a dupe...

George W. Bush isn't precisely a junior, but reversion to the mean has worked with a vengeance in his case. The end result will be that history will upgrade his father to “the good George Bush” to distinguish him from his successor and namesake. Brit Hume was a sucker for a weakling with a good pedigree twenty years ago, and he remains one today. As a regular apologist for Bush administration pratfalls, Brit was the perfect choice for Dick Cheney's lukewarm and muddled apologia for his clumsy shooting of a hunting companion. The only damage Cheney took from his interview on Fox stemmed from his general incoherence rather than Hume's close questioning. (How many of Brit's questions were written for him by White House flacks?)

Not to belabor the point, I would like to share some of Brit's comments from Living with jr in back issues of the Capital PC Monitor. His talent for making excuses in the face of incompetence and disappointment goes back at least that far.

Take heart, PCjr owners. IBM's loss can be your gain. Contrary to what you may have read about the jr, it has turned out to be one of the great values in the history of microcomputing. (December 1985)

---

And George W. Bush will turn out to be one of the great presidents in the history of the republic.

There are a lot of ways to expand a PCjr, but if you aren't careful, you can end up spending as much as the cost of the computer itself, or more. (January 1986)

---

“Or more”—the mantra of Bush policy toward tax cuts and defense spending, both of which enrich his political allies.

Few people can enter the basement room that houses my home computer system without gasping at the rat's nest of wires that hangs from the rear of it. This is not what IBM had in mind when it designed the PCjr.... (April 1988)

---

All computers are festooned with wires and cables, but it's worse when even basic equipment needs to be installed as add-ons. Making the jr into an adequate computer frequently involved the purchase of a second entire chassis to install the expansion boards that would not fit into the original system unit. I suppose the obvious parallel to try to draw with the Bush administration is to suggest that we need a second president to offset the inadequacies of the one we have... But we tried that with Cheney, right? And we all know how that is working out.

Note: The good old days of personal computer user groups are behind us now. Except for rare survivors like the Houston Area League, most of the clubs are gone or reduced to shadows of their former selves. I joined a number of these groups just to get their excellent newsletters. The Monitor was a prime example. My archive of its back issues probably makes me one of the few people on the West Coast who could have written this article. Once again procrastination in cleaning off the shelves has proved its worth!

Prestidigitation

Nothing up my sleeve

Oh, yeah, this is me, all right. The hands tell a story. If you turn off the sound, my lectures would be pantomime theater. Who knew this was state of the art instructional practice? I thought it was because I'm Latin.

Lesson plans & pans

Inmates and asylums

It's a very ancient saying,
But a true and honest thought,
That if you become a teacher,
By your pupils you'll be taught.

The King and I, Oscar Hammerstein II

---

Like most of my colleagues in the teaching profession, I don't really think that I have all of the answers. In general, though, I'm pretty sure that I have more of them than my students do. One of teaching's most fascinating aspects is the teacher's constant interaction (and occasional combat) with the many-headed beast known as the classroom. After a couple of decades, I think I've met most of the student archetypes, but there's still room for the occasional surprise.

Several years ago, a calculus student patiently explained to me that he did not need to do homework. After all, if I did my job right and provided good examples in class with lucid expositions, he would understand the concepts and procedures of the subject without any of that tedious grubbing about with homework problems. I heard him out as patiently as I could. He really seemed serious about it, although I had entertained the possibility that he was a more subtle joker than I usually encountered. I asked him if he could ride a bike. He thought about that for a while, seemed to get the point, and didn't press his argument.

Another student habitually made many small errors on every quiz or exam, losing lots of points for forgetting basic steps or using formulas incorrectly. When we discussed his situation, I suggested he take more time working on the notecard I permit my students to use during exams and quizzes. (I do not emphasize memorization, but prefer to reduce test anxiety by allowing students to refer to a handwritten notecard. Some of them realize that they actually learn the material while poring over their text and notes for material to write on their cards. I do not always warn them about the potential for inadvertent learning.)

My student explained to me that he did not have a notecard. That was unfortunate, I replied, because he had lost several points by confusing the point-slope form of a linear equation with the slope-intercept form. If only he had written them down and labeled them properly, he would not have gotten as confused during the exam. He agreed that I had a point, but he informed me that it was not right to use a notecard during exams because that was cheating. “It can't be cheating if you have your instructor's permission. It's cheating only if a notecard is not allowed and you use one anyway,” I explained. No, he insisted, he just didn't feel right about it. I agreed it was his choice. Later, after complaining that I expected them to remember an unreasonable amount of material on each exam, he dropped the class. Oh, well.

The boycott approach

This semester I am experiencing something new in student behavior. It's not uncommon for students to complain about some aspect of a course and express a wish that it were different, but one of my elementary algebra students has gone quite a bit further. She has adopted the novel approach of boycotting the class until I mend my ways. As you might imagine, I feel an intense pressure to capitulate so that this particular disagreeable student will once again grace the class with her presence. She has been sending me daily e-mail dispatches from her stronghold (the college library) while waiting for me to snap.

I am in the library using the time constructively to move myself ahead so I will no longer feel like I am being pushed by your incessant daily quizzes.

---

My algebra class stumbled rather badly on the second exam of the semester, which was devoted to linear graphs and their equations. Since my students will be doomed in intermediate algebra if they don't master linear equations in elementary algebra, I recycled some of the exam problems into quizzes that I give each day while continuing to progress through the syllabus.

I simply feel it would be more constructive if instead of so many quizzes, more time was spent reviewing the homework problems in preparation for WEEKLY quizzes and the tests. It has only been one month since this semester began. Already there has been 10+ quizzes. Quizzes that have achieved little more than an approximate 50% failure rate.

---

The “10+” is strictly correct: There have been 11. However, she exaggerates the failure rate on the quizzes. I wish she had noticed that she was doing better on the quizzes than on the exams. I posted averages for each.

Instructing on the current daily homework lesson problems before the homework is done, then going over the problems in class the next day would do alot [sic] in promoting student/teacher relations. What goes on in class is a rapid-fire hit-and-miss construct of the homework that has already been assigned leaving no time for instruction on the current daily homework assignment and review. It is like putting the cart before the ox.

---

While that's an interesting variation on the theme of carts and horses, the curious fact is that my class periods routinely begin by my taking questions on the previous day's homework assignment. Until she began boycotting the class, my unhappy student was frequently the first person whose question got answered. And so far this term I've been pretty successful at covering all the new topics each day, complete with worked examples. Oh, well.

More emphasis should be put on helping students understand the current daily homework assignment problems than on your perceived goal of higher daily quiz grades. The grades you posted for the two classes you teach show approximately half of the students in each class FAIL. The only constant in those statistics is the common factor of having the same teacher; the same instruction construct.

---

She exaggerates the proportion of failing students on the roster, but I'm glad she picked up on my goal of getting their quiz scores higher. Frequent quizzes are a great device for determining whether students are staying caught up with the material (and I've learned that some of them are decidedly not), as well as an effective means for letting students know what to expect on exams. The exams are weighted much more heavily in the grading system outlined in my syllabus, and I pointed out to my students that the low weight of the quizzes means their mistakes on initially encountering quiz problems can be offset when they meet similar problems on the tests.

Perhaps some serious consideration should be given to reconstructing instruction techniques that achieve greater student understanding of the daily homework problems in preparation for tests, improving student/teacher relations, than attempting to achieve statistics using problematic methods.

---

My student's writing is fairly competent and peculiarly euphonious. I've written back to thank her for her comments and to explain that (a) the class is not going to be restructured to suit her preferences and (b) the one certain result of not coming to class is a failing grade. Some interesting thought processes are going on here and I confess that I do not understand them. I wish I did.

Who is Zeno?

Persistence & patience

“Zeno” has been my on-line handle for several years, dating back to the era when electronic bulletin board systems (BBSs) were popular among computer hobbyists. These systems were the forerunners of today's websites and blogs. Users would log on to their favorite BBS to read and post messages, both public and private. Some BBSs offered live chat and on-line games, while others specialized in supporting particular products or organizations. The computer club I belonged to had its own BBS for the dissemination of meeting announcements and user-supported software.

Most BBS users favored anonymous handles instead of their real names, both for convenience and personal security—exactly the same reasons as today for pseudonymous e-mail addresses and user names. I no longer recall exactly how I picked “Zeno” for my handle, but the choice made so much sense that it's stayed with me over the decades. There is more than one Zeno in history that figures into my well-suited pseudonym.

Zeno of Elea

I encountered Zeno's paradoxes early in my career as a math major in school. These conundrums were devised by Zeno of Elea (or “Zeno the Eleatic”) to provoke his fellow philosophers. Zeno and his contemporaries of the fifth century B.C.E. did not have a good formulation of the notion of infinity, which permitted Zeno to stir up trouble. He famously argued that even a great athlete like the warrior Achilles could not win a foot race with a tortoise if the tortoise were given a head start. After all, by the time Achilles ran up to the tortoise's starting point, the tortoise would have moved to a new location further down the track. We are now back where we started from! Achilles is running a race with a tortoise that has a head start. He must run to the tortoise's new location, by which time the tortoise will have moved on again. Repeat. Repeat. Repeat ad infinitum. Obviously the great athlete never overtakes the plodding terrapin.

Except that in real life he does, hence the paradox. Zeno challenged his fellow philosophers to find the flaw in his argument, which they could not adequately do because they recoiled at the notion of infinity. Today we have a much more robust notion of infinity (we know, in fact, that infinities come in different sizes!). It's even built into our system of mathematical notation: the decimal number system.

Even in simple arithmetic, students meet infinity as soon as they begin to work with decimal fractions. They learn that 0.33333... is the decimal fraction corresponding to 1/3 and we teach them techniques to switch between decimal and ratio form. In later mathematics, after we have worked out the properties of geometric series, we see how an infinite collection of numbers can have a finite sum. A good example is 1/3 = 0.3 + 0.03 + 0.003 + 0.0003 + ....

As a teacher, I like to illustrate Zeno's paradox by claiming to my students that no one can leave the classroom. Of course this catches their attention. I tell them to consider that they have to get to the door in order to leave the room. However, wherever they are in the room, they first have to travel halfway to the door. From that point, they have to travel half of the remaining distance. Repeat ad infinitum. To actually reach the door, each student is accumulating a sum of distances: 1/2 of the original distance, then half of what's left (which is 1/4 of the original distance), then half of what's left of that (1/8 of the original distance), etc. What do we get when we try to evaluate the sum 1/2 + 1/4 + 1/8 + 1/16 + ...?

We know from everyday experience that we really can get out of the classroom, so what's going on with the “halfway there” argument? If we revisit the process of successive halves, we notice that first we travel 1/2 of the distance, followed by 1/4 of the distance, putting us 3/4 of the way to the door. The next stage is 1/8 of the distance, so 3/4 + 1/8 = 7/8. If we continue adding the individual stages and considering each result, we progress to 15/16 of the distance followed by 31/32, 63/64, 127/128, etc. These numbers are tending toward 1, the entire distance to the door, a finite number. By breaking up the distance into an infinite number of segments we make it appear that it is impossible to escape from the classroom, but by considering the sums we observe that the distance is finite and not the insurmountable barrier we feared.

While some students will remain perturbed by the problem, I tell them it should not embarrass them to be puzzled by a conundrum that used to defeat the best minds of Zeno's day. While this discussion by no means exhausts the topic, it illustrates why I am fascinated by Zeno's paradoxes and borrowed his name for my on-line handle. But while Zeno of Elea appeals to my mathematical side, another Zeno appeals to my philosophical side.

Zeno of Citium

The philosophical school of stoicism is named after the Greek word “stoa” for porch. Zeno of Citium (333 B.C.E.-264 B.C.E.) taught his system of thought from his eponymous painted porch. While porches are out of favor in modern homes, we've all seen that porches are a great place to take it easy. I think it's quite remarkable that Zeno's fame is based on hanging out on the front stoop and shooting the breeze with his buddies. It reminds me of Douglas Adams's characterization of a philosopher from Hitchhiker's Guide to the Galaxy: “Trin Tragula ... was a dreamer, a thinker, a speculative philosopher or, as his wife would have it, an idiot.” I don't know whether Zeno of Citium had a wife to rail against him while he expounded on topics philosophical, but I do know that his school of philosophy has left us with a useful adjective: stoic.

I admire the stoic outlook, although I cannot claim to be particularly good at emulating it. Zeno taught that personal tranquility could be attained by a serene indifference to pleasure or pain. That seems to make sense. The devoted stoic steels himself against perturbations of the spirit by even-handedly disdaining the pursuit of pleasure and armoring himself against pain. This is no doubt what Lennon and McCartney were warning against in Hey, Jude (“Well don't you know that it's a fool who plays it cool by making his world a little colder”). Still, serenity is a pearl of great price, so who can say what someone might pay for it? Zeno is said to have lived an ascetic life, so perhaps he achieved the tranquility he sought. In general, as a fretful and compulsive worrier, I would have to say that I have not. Yet one could do worse than be an aspiring stoic.

Still other Zenos

The thinkers of Elea and Citium by no means exhaust the list of history's Zenos. Check out this Wikipedia page for references to several others. As for today, Zeno is variously a rock band as well as an aspiring model of Italian and Puerto Rican descent. I probably should add that Nikki Zeno (Lowrider cover girl for March 2003) is much too young to have influenced my choice of pseudonym twenty-five years ago and her views on mathematics and philosophy are not an inspiration for the content of this blog. Just thought you should know.

Divine inspiration

God is kitsch

The California high school exit exam has started to exact its toll on students who now face the possibility of ending their senior year without a diploma to show for whatever effort they put in. No doubt this has lead to higher levels of prayer in school, which—despite the propaganda of the Christian right—has never been banned from public education. (Students can pray whenever they like as an act of personal freedom, but it's not your teacher's job to lead you. Simple as that.)

For many years religious conservatives have been a major part of the back-to-basics movement. Those who haven't fled to home-schooling want public schools to drill students relentlessly on the classic three R's. They are all in favor of rigorous standards and strict accountability.

As a math teacher I am accustomed to maintaining pretty strict standards with my students. Nevertheless, I account myself a skeptic of the back-to-basics movement, the push for high-stakes testing, and the resurgence of rote learning. It strikes me as a reactionary campaign to return to a past that never really existed, when “all” students were eager and successful learners—well, at least those who were in school in those days instead of in the fields and factories.

Still, it had not occurred to me until recently to question the sincerity of those who argue the fundamentalist educational cause. But it turns out that some of these rigorists are eager to make excuses or exceptions for their fellow travelers. I am not talking about smarmy little prevaricators like the recently fired NASA public information employee who lied about his college drop-out status while injecting his sectarian point of view into government research on the Big Bang and the age of the universe. No, my epiphany came during EWTN's Open Line broadcast last Tuesday. It turns out that the most miserable dreck passes for divinely inspired art when churned out by one of their own.

On Tuesdays it is Barbara McGuigan's turn to host Open Line. I've mentioned her before. Her shtick is pro-life activism. McGuigan likes to encourage her listeners to lobby against abortion without fear of ineloquence because God “gives us the words to say.” This past week, on February 7, a caller named Theresa shared with Barbara a poem she had written, no doubt under divine inspiration. Brace yourselves, folks. Here is the whole thing:

The hardest choice

Did you buy that lie?
It's not just an apple anymore.
We've turned it into a pie
Overflowing and growing with sweet knowing
In which the words are changed and all rearranged.

So do you get this trick on the rhetoric?
Don't buy a pie with a lie.
Get a pie with no lie,
One where all is growing with humble, meek knowing
With grace from above that comes with selfless love.

So never quit when you find you're not fit
And don't fall apart, just open your heart.
Get into the right story that's filled with mercy and glory
For you, for me, for all.

So choose to seek and live the wisdom from above
On the way to the kingdom of love.

---

McGuigan was delighted with the poem and squealed, “That's beautiful, Theresa! I love it! That's wonderful. Wonderful poem!”

A few possibilities suggest themselves. Perhaps Barbara was merely being polite (I doubt it). Maybe Barbara has a tin ear (quite likely). Possibly Barbara gives high marks to anything—no matter how dreadful—that follows the party line (Bingo!). It was a moment of cognitive dissonance.

I listened to the recording of the poem several times while transcribing it as accurately as I could for your edification. Several times. Please pray for me!

Postscript: I regret to inform you that the figurine of Jesus playing football is part of a series.

Boulder in the sky

What goes around

Look at the moon. I mean really look at the moon. Crane your neck and regard the earth's traveling companion. Try it. Were you stunned by what you saw?

You should be. Unfortunately, the moon is to most of us just an everyday object that recedes into the background. That describes my usual reaction to the moon, too. However, I am a child of the Space Age who was a teenager at the time of the first moon landing. When I dig back into my memories, I recall how it seemed at the time. On occasion, I can still recapture some of those sensations.

It happened again recently. The moon helped. I was out for a late afternoon stroll and a dab of white in the sky caught my eye. The timing was perfect. The sky was blue and clear; the waxing moon was slightly past half full. And it was round. The moon does not always strike the eye as a round object, but a couple of days before or after the half moon is the perfect time to capture the moon's three-dimensionality. The terminator—the line separating the lit side of the moon from the dark—is gently bowed at those times, turning the moon's visual aspect into a ball. Half moon is no good. The terminator is a straight line right down the middle and all you see is a half-disk in the sky. If anything, the full moon is worse. The washed-out satellite turns into a tarnished silver disk mounted on a sable background. The full moon has no more depth or mystery than an Elvis on velvet.

I, on the other hand, looked up at the moon at the perfect moment and saw a huge round rock hanging in the sky. When this perspective seizes your senses, you are suddenly on the greatest thrill ride in the world. There is a huge rock hanging in the sky! And the earth beneath you also becomes an orb, a sphere on which you are standing. Try not to get too dizzy. There is no better moment at which to capture the sensation of living in a planetary system than when you look at the partially lit moon with the understanding that you are standing on a similarly lit sphere.

After some seconds—maybe a couple of minutes if you're lucky—you come back to earth and the moon is just the moon again. Some little-regarded reflector of the sun's glory. But you'll remember that you had the sensation, even if the sensation itself is gone. And one day it will all strike you again.

You are here

I am not a traveler. While I don't mind seeing new places, I find the process of getting there too onerous to endure cheerfully. Airports and security screenings particularly put me off. Any trip that takes me too far away to end up spending the night in my own bed is normally too long a trip as far as I'm concerned. I know that most people are not homebodies to the degree I am, but that's the situation.

Nevertheless, I have been overseas. Once. I travel a few hundred miles every couple of months to visit family (both of my parents are alive and in good health). And recently I was talked into going to a conference for math teachers in southern California. It was sponsored by my publisher and I resolved that it would be a good thing to do. It was. I met some excellent math professors from several western states, had great discussions with them, and enjoyed the off-season resort locale.

I also noticed something I had forgotten about previous trips: Travel really is distracting. Problems that previously obsessed you seem to recede into the background and you end up regarding them from a new perspective. Perhaps you even discover that what you thought was serious is rather trivial. Breaking out of a routine can shake things up and recharge your batteries. I guess that's what people mean when they talk about vacations and recreation. I'll have to remember that.

I am, of course, constructing a parallel here. You don't actually go anywhere when you look at the moon. (Remember, I mean look at the moon.) But it can give you a tiny bit of perspective that reveals the scale at which we live. Whether that pleases or daunts you is an individual reaction.

My nephew and I were discussing the moon the other day. He informed me that you have to wait till night to see the moon. He's six, so he's not to be judged too harshly for his observational lapses. Many adults probably think the same thing. The moon is merely more obvious during the night, but it spends as much time above the horizon in daylight as it does in night-time. It's always there, visible more often than not, ticking away the clockwork of the spinning system in which we reside.

It is an extraordinary occurrence for a planet to have a satellite as large as our moon. Sure, the moons of Jupiter are even larger, but they shrivel to insignificance next to the bulk of Jupiter itself. Proportionately speaking, our moon is an enormous planetary satellite. It is both large enough and close enough to be a spectacular sight in earth's sky—a spectacle to which we have become accustomed. Try, however, to look up at it with new eyes. And brace yourself.

War is money

Commander Cuckoo-Bananas

Iraq is not Vietnam. It is worse. When North Vietnamese forces marched into Saigon in 1975 and renamed it Ho Chi Minh City, the result was a united Vietnam that had little or no power to harm significant American interests. By contrast, when our actions turn Iraq into an Islamic republic allied with Iran, we will have created a new monster in place of the one that was already there. And it will be rich with the oil we crave.

The despotic Saddam regime was a secular institution whose numerous atrocities were based on power politics rather than any religious ideology. We were willing to look the other way for many years while Iraq was one of our client states in the Middle East. The U.S. showered Saddam with attention and visits by such high-ranking government officials as Donald Rumsfeld. Then Saddam made his ill-advised incursion into Kuwait and we suddenly noticed he was a bad guy.

The fall of a cruel dictator should always be occasion for celebration, but the Bush administration's incompetent handling of the short, glorious war (now approaching the end of its third year) has plunged us into a morass of our own making. Iraq is now a pesthole of insurgency, terrorist strikes, and suicide bombings. Our troops are not being showered with flowers. Even our chronically dishonest Commander-in-Chief has admitted that 30,000 Iraqis have died as a consequence of our invasion of their country (it's probably significantly higher). At this time, American fatalities are edging past 2500, while non-fatal injuries are much greater than that. Our soldiers were sacrificed to settle George W. Bush's grudge against Saddam Hussein and to permit the president to portray himself as a greater warrior than his father, who permitted Saddam to remain in power after the first Gulf War.

And then there's the money.

We are pouring money into Iraq with a fine disdain for such details as record-keeping and accountability. No-bid contracts are bestowed on Bush's business sector allies, notoriously including such amoral gougers as Halliburton. In addition to the over-billings for which these contractors are famous, they are wondrously in tune with the Bush administration's tradition of incompetence. (See, in particular, Tim Lambert's observations on our inability to provide Iraq with better energy supplies.)

We pay huge amounts to contractors to perform tasks that could be performed much more economically by troops we already have in place. Are the expensive contractors in Iraq being used to free up more of our over-extended troops for military action? No, that would make too much sense. Instead our troops end up babysitting the contractors.

This is not idle speculation on my part. It comes directly from Iraq via the observations of an Army captain who is frustrated by the abuses he sees every day:

[T]roops are deployed and providing redundant support backing up civilian contractors. This is what is happening to my unit.... At 3 of 4 points we are providing support that the civilian contractors, which are already on site, are capable of providing. As a taxpayer I think this is a terrible waste of our tax dollars. The DoD pays those contractors outrageously to ensure they do not fail, and then we put soldiers next to them to double ensure they don’t fail. What a waste—not to mention putting troop in danger needlessly.

---

The disgruntled captain is a career soldier whom I have known for many years. He hopes that American citizens will take more seriously their right to call their government to account:

I am glad to hear people are starting to publicly take Bush to task over his conduct of the war in Iraq. I felt that in the past he hid behind soldiers by saying that anyone critical of the war didn’t “support the troops.” I honestly feel great support from most Americans, and I think there is nothing more American then questioning the policies and techniques for our government. We should never forget the philosophies that drove our forefathers to rebel against the English Government.

---

The captain is particularly concerned that Bush's “Great War on Terror” seems to be a mission without a goal or definition. He is accustomed to following orders with content and specificity. He lapses into Army jargon when describing his concerns:

As I hear the arguments I come with several questions I would like answered. One of the Principals of War is to have a clear, definable objective. What is our clear, definable objective in Iraq and the Global War on Terrorism? Bush's benchmark of an Iraqi government that can maintain stability is vague and easily re-definable. What forces are actually required in theater? Not a list of just the Brigade Combat Teams (BCT), but a carefully analysised list by Table of Organization and Equipment (TOE) or Modified Table of Organization and Equipment (MTOE) of currently required forces. MTOEs (which we pronounce emm-toe) give each unit our mission and authorize troops and equipment. Making the CENTCOM justify each and every MTOE they want to continue the fight is only way we will know who can go home.

---

And when.

The captain is concerned that the war is being conducted by amateurs, ideologues who try to force reality to conform to their astigmatic vision rather than bothering to comprehend how things really are. He was impressed with the effectiveness of the initial invasion plan that allowed a light-weight American force to topple the Saddam regime in a matter of days. He despairs, however, that anyone has the vision to craft a rational post-Saddam program and withdrawal schedule. It may even be too late to salvage American credibility now that Iraq is embroiled in what amounts to a civil war. What are we really doing there now?

The comments that I quoted from my friend the captain were contained in an e-mail message he sent to me in early December of last year. He told me I was free to share his observations, but to please leave out any details of his posting or identity. He knows that a member of the armed forces cannot criticize the Commander-in-Chief in public and he did not want to get in trouble because of a private message to a friend.

But I wonder. I have not heard from him since he wrote that e-mail more than two months ago. And I worry about him every day.

The naked student

Empty-headed & empty-handed

The student came up at the end of class to ask me a question. He looked vaguely familiar, which is quite unusual. It's the end of the third week of the semester, by which point I recognize all of my students and know them by name. Another unusual factor: The student was academically naked. No, I don't mean anything that might violate the public decency standards. I mean he was completely unencumbered by anything that might tip one off to his student status. He carried no textbook, no notebook, no backpack, no calculator, no pen or paper—not even a sheet of paper. He had as much academic paraphernalia as the day he was born.

His question was unusual, too:

“Am I in your class, Dr. Z?”

“I don't know,” I replied. “Who are you?” He told me his name and I checked my grade book. He wasn't listed on my current roster, so I paged back to an earlier print-out. “Oh, there you are. I dropped you.”

He seemed surprised, even though his question suggested he had anticipated the answer. He had a follow-up question:

“Can I do anything to get back into the class? Could you reinstate me?”

“Why would you want me to do that?” I asked.

My reply stumped him for several seconds. I followed up: “We have been in session for three weeks. You did not show up for the exam we had on Monday. You were late this morning, so you missed the quiz I gave at the beginning of the period. In fact, you've missed all five of the quizzes we've had so far. You didn't even manage to do the very first assignment of the semester, which was to e-mail your instructor during the first week. I don't have a single point recorded for you in the grade book, so of course I dropped you.”

He pondered. I suggested he consider signing up for the class when he was actually planning to take it. He thanked me for my advice and strolled off.

It was a teachable moment.